"Also, who the shooting back will do?" part II

Yesterday I ran some numbers on the ever-growing disruption ball weapon featured in Ralph Roberts' "The Gun That Shot Too Straight" (Dragon #94, February '85). My calculations indicated that the fireball shot from Henry's pistol travels at 350 meters per second and grows at .03 millimeter per second. Today I'm going to apply those numbers to some astronomical targets.

First up on the hit list is the Moon, which is usually something around 384,399 kilometers away from us. Looks like it will take about 13 days for the fireball to reach ol' Luna. The entry wound on the Moon will be about 37 kilometers in diameter. I actually think that might be visible from Earth, as 37km is in the neighborhood of 1% of the Moon's diameter. Here's a rough mock-up:


The fireball will take about 2 hours and 45 minutes to drill through the Moon, assuming the shot is angled to cross the centerpoint of the sphere. Time to aim the disruption pistol at our next victim, Mars.

I probably don't need to tell you that Mars is a LOT farther away than the moon. Since both Earth and Mars orbit the sun at roughly similar distances, the distance between the two planets varies greatly. We'll use the middle range number of 1.5 Astronomical Units, or about 225 billion meters. Our slow-moving sphere of doom will need a tad bit more than 20 years to reach the Red Planet. Uh, oh! Mars is in BIG trouble because at that point the disruption energy is about 21,000 kilometers in diameter! The encounter between Mars and the disruption sphere would look a little something like this:


So Mars is a complete goner and somewhere along the way the disruption effect becomes a visible astronomical object. The initial firing of the weapon produces a light described as "searing bright", so it may be visible on its way to the Moon.

About 50 years or so later the great glowing sphere of doom will reach approximately the orbit of Jupiter. Assuming its flightpath intersects with Jupiter, the resulting annihilation isn't quite as absolute as poor Mars, but Jupiter is still pretty effectively destroyed. I don't see how the planet is going to keep it together in any recognizable form wearing a hole this size:


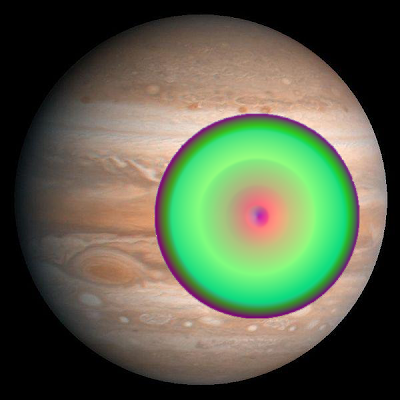
(I think this point is as good as any to note that I've been assuming all along that the sphere of disruption has no mass .)

Four and a half centuries from the time the trigger is pulled on Earth the deadly orb will reach the neighborhood of Pluto. I'm not going to even bother with a graphic of Pluto's destruction here because the diameter of the disruption sphere will have grown in size to half a million kilometers, or more than the distance between the Earth and the Moon. They wouldn't be anywhere near each other, but here's a scale comparison between the Earth/Moon system and the disruption field circa 2435AD:


The next target on the celestial hit list is the Alpha Centauri system. At roughly 4.3 light years away the fireball (still assumed to be traveling only 350 m/s) will take a helluva long time to reach its destination. Roughly 3.7 million years, as a matter of fact. Even growing at a measly .03 millimeters per second, that allows for expansion to 3.9 billion kilometers in diameter, or about 26 Astronomical Units. That means that when the fiery ball o' doom reaches Alpha Centauri it's almost as big as what we normally think of as the Solar System (i.e. the Sun and its planets). If a disruptor ball that size passed through the Solar System on a course centered on the sun everything inward of Neptune would be toast. As you can guess, Alpha Centauri would be in a world of hurt as well. The Alpha Centauri twin stars orbit each other at a distance of about 17 AU, so both could be completely consumed by the fireball. At least if we assume that the 'molecular disruption' effect of Henry's pistol would have an effect on stellar material. Distant companion Proxima Centauri would be safe.


Finally, let's aim this puppy at the Andromeda galaxy and see what the numbers have to say. Andromeda is pretty close galacticly speaking at only 2.5 million light years away. That is a quite a distance to travel at only 350 meters per second. My back-of-envelope estimation puts the time to target at 2,151,162 million years. Not 2 million years, 2 million million years. Under the American numeric system I believe this would mean 2 trillion years.

Over that long of a time scale I have my doubts that the Andromeda Galaxy as we know it would exist to blast, as 2 trillion years from now is over 140 times the present age of the universe. Instead of the present Andromeda Galaxy, when the doomsphere arrives there might only be a thin cloud composed of dim objects like red dwarfs, white dwarfs, brown dwarfs, and black holes. Aiming Henry's pistol at the Andromeda Galaxy would in effect be putting out a hit on a 95 year old man on life support. Just not much point to it.

Of course the big final question is how big the fireball grows over 2 trillion years. The answer is a whopping 2.3 quadrillion kilometers, or 15 million AU, or roughly 244 light years across. Which brings us back around to a case somewhat similar to the Moon above, where the diameter of the fireball is equal to roughly 1% the width of the target. Of course galaxies even in the present era are mostly empty space, so the ravaging ball of disintegrating energy would probably pass right through Andromeda with no discernable effect. Future Andromeda will be even more insubstantial.


Well, I think that's enough slapdash math, crappy graphics, and cosmic destruction for one day.